Question

TRIGONOMETRY what is the angle of the person’s resultant vector?

Answer 1

Using the data statement, we plot the following diagram:

We see that the angle of the result vector is:

`\theta_1=180\degree+\theta_2\text{.}`

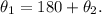
The second angle can be computed using the trigonometric relation:

`\tan \theta_2=(OS)/(AS)\text{.}`

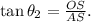
Where:

• OS = opposite side to θ_2 = 780 m,

,

• AS = adjacent side to θ_2 = 360 m.

Replacing these values in the formula above, we have:

`\tan \theta_2=(780m)/(360m)=(13)/(6)\Rightarrow\theta_2=\arctan ((13)/(6))\cong65.22\degree.`

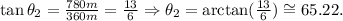
So the resultant angle is:

`\theta_1=180\degree+\theta_2\cong180\degree+65.2\degree=245.2\degree.`

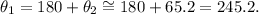
Answer

The resultant angle is 245.2° to the nearest tenth.