Question

How many solutions does this system of equations have? Explain your reasoning.

Answer 1

We are given the following system of equations.

`\begin{gathered} 9x-3y=-6\quad eq.1 \\ 5y=15x+10\quad eq.2 \end{gathered}`

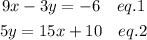
We are asked to determine how many solutions does this system of equations have

Let us solve the system of equations to find out.

Let us use the substitution method.

Separate out one of the variables from any of the two equations.

Let us seperate out variable y from eq.2

`\begin{gathered} 5y=15x+10 \\ y=(15x+10)/(5) \\ y=(15x)/(5)+(10)/(5) \\ y=3x+2 \end{gathered}`

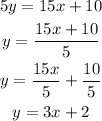
Substitute this value of y into eq.1 to find the value of x.

`\begin{gathered} 9x-3y=-6\quad eq.1 \\ 9x-3(3x+2)=-6 \\ 9x-9x-6=-6 \\ -6=-6 \end{gathered}`

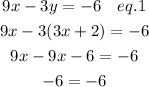
Notice that the variable x got canceled out and the equation is satisfied (-6 is equal to -6)

This means that there are infinite solutions to the given system of equations.