Question

a rectangle garden has a width of 90 feet. the perimeter is 500 feet. what is the length of the garden? 500=(2×l)+(.....×.... ) 500=2l+...............=2l.........÷2=l........=l

Answer 1

Given

A rectangle garden has a width of 90 feet. the perimeter is 500 feet.

We are to solve for the length of the garden

`The\text{Perimeter of a rectangle =2(L+W)}`

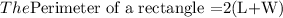
Where L is the length and

W is the width

From the question

W = 90 feet

P = 500 feet

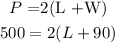
`\begin{gathered} P\text{ =2(L +W)} \\ 500=2(L+90) \end{gathered}`

Another way

`\begin{gathered} P=2(L+W) \\ P=500ft\text{ and W=90ft} \\ 500=(2* L\text{ )}+(2*90) \\ \\ 500=2L+180 \\ \text{collect like terms} \\ 500-180=2l \\ 320=2l \\ \text{divide both sides by 2} \\ (320)/(2)=(2l)/(2) \\ \\ 160=l \end{gathered}`

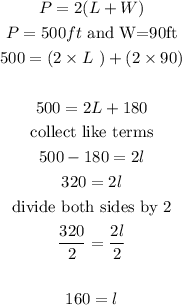
The length of the garden is 160 feet