Question

A basketball court is in the shape of a rectangle that is 94 feet long and 50 feet wide. What is the length of a diagonal of the court? Round to the the nearest tenth.

Answer 1

104.5 feet

Explanation:

Given a basketball court in the shape of a rectangle with the following dimensions:

• Length = 94 feet

,

• Width = 50 feet

The diagonal of the rectangle divides the rectangle into two right triangles as shown in the diagram below:

The length of the diagonal forms the hypotenuse of each of the right triangles.

Using Pythagoras' theorem, we find the value of the hypotenuse labeled x above:

`\begin{gathered} x^2=94^2+50^2 \\ \text{Take the square root of both sides:} \\ √(x^2)=√(94^2+50^2) \\ x=√(11336) \\ x=104.5\text{ ft} \end{gathered}`

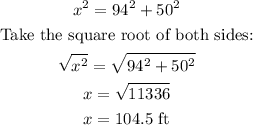
The length of the diagonal of the court is 104.5 feet (rounded to the nearest tenth).

own in