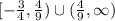Given the function:
![h(x)=\frac{6\sqrt[]{4x+3}}{9x-4}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/2doyb7erg6sa5mku8u8c.png)
Let's find the domain of the function.
The domain of a function is the possible values of x which makes the function true.
To find the domain of the function, set the denominator to zero and solve for x.
We have:
9x - 4 = 0
Add 4 to both sides:
9x - 4 + 4 = 0 + 4
9x = 4
Divide both sides of the equation by 9:

Also, set the expression in the radicand greater or equal to zero and solve for x.
4x + 3 ≥ 0
Subtract 3 from both sides:
4x + 3 - 3 ≥ 0 - 3
4x ≥ -3
Divide both sides by 4:
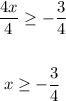
The domain of h is:
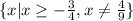
Therefore, the domain of h in interval notation is: