Given:
The given roots are -2, 5, and 7.
To find: Write the polynomial equation of the least degree with roots -2, 5 and 7?
Step-by-step explanation:
We have given that
Given roots of polynomial equation are -2, 5, and 7.
The least degree polynomial equation will be
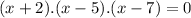
We solve the above expression we get,
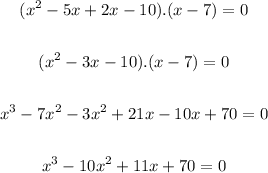
Hence, the least degree polynomial equation is x^3-10x^2+11x+70 = 0.
Answer: The least degree polynomial equation is x^3-10x^2+11x+70 = 0.