We are given an equation to model the population of a bacteria after t hours with the following form:
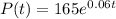
By replacing 0 for t we determine the population of bacteria at the begining of the experiment, then we get:

Then P(0) = 165, this means that at the begining of the experiments there were 165 bacterias.
In order to determine the population after 5 hours we just have to replace 5 for t into the model, then we get:
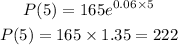
Then, the population of bacteria after 5 hours will be 222. Similarly for 10 hours:
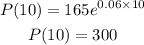
Then the population of bacteria after 10 hours will be 300