Solution:
Given:
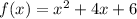
To approximate the definite integral using given partition and sample points, we use the formula

From the interval given,

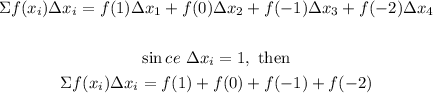
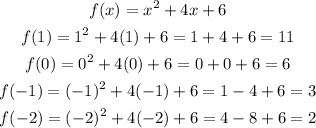
Hence, the approximate value of the definite integral is;
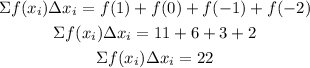
Therefore, the approximate value of the definite integral is 22.