Question

The diagram shows a circle centered at the origin. A right triangle is located in quadrant 2 as shown.Write expressions for the sine and cosine of the angle marked 0. Is the sine positive or negative? Is the cosine positive or negative? Explain how you know. Type your answer in the box below

Answer 1

The sine function is given by:

`\sin (\theta)=(opposite)/(hypotenuse)`

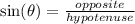
Therefore:

`\begin{gathered} \sin (\theta)=(y)/(r) \\ (y)/(r)>0 \end{gathered}`

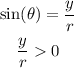
Therefore, we can conclude the sine is positive in II quadrant.

----------------------------------------------------------------

The cosine function is given by:

`\cos (\theta)=(adjacent)/(hypotenuse)`

therefore:

`\begin{gathered} \cos (\theta)=-(x)/(r) \\ -(x)/(r)<0 \end{gathered}`

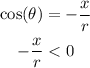
Therefore, we can conclude the cosine is negative in II quadrant