Question

Solve the system of equations by graphing.If there is a unique solution, write the solution as an ordered pair.

Answer 1

GIven:

The given system of equation is,

`\begin{gathered} 2x+3y=-23\ldots(1) \\ x+3y=-19\ldots(2) \end{gathered}`

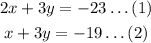
To find: Solve the system of equations by graphing.

Step-by-step explanation:

we have to graph the both the equation the graph will intersect at one point and that point will be the solution to the given system of equations.

First let us graph the equation (1),

The graph of the equation,

`2x+3y=-23`

Now, graph the equation (2),

The graph of the equation,

`x+3y=-19`

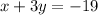
now, let us combine the both graph and check the intersection point for the solution.

The graph is,

The intersection point is (-4,-5)

Final Answer :