Question

Richard has two different plans for his business. Plan 1: He charges $3 per hour to walk a dog plus $10 for grooming at the end of the walk. Plan 2: He charges $5 per hour to walk a dog and then offers free grooming at the end of the walk. Part A Write an equation to model the time in hours, h, when the two plans cost the same amount. Part B When will the two plans in hours, h, cost the same amount? h =

Answer 1

First, write an equation for the amount of money he wins for each plan, given a time of h hours:

`\begin{gathered} P_1=10+3h \\ P_2=5h \end{gathered}`

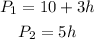
If both plans cost the same amount, then P_1 = P_2, therefore:

`10+3h=5h`

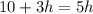
Solve the equation for h:

`\begin{gathered} \Rightarrow10=5h-3h\text{ } \\ =2h \\ \Rightarrow h=(10)/(2) \\ \Rightarrow h=5 \end{gathered}`

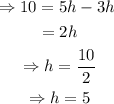
Therefore, for Part A the equation is 10+3h=5h

For Part B, the value of h when both plans cost the same amount is h=5.