Question

Use the given cost tables for the same product from two different companies to create alinear system. Then solve the system to determine when the cost of the product will be thesame and what the price will be.Letr(n) represent the cost of paper towels at Restaurant Warehouse and let s (n) representthe cost of paper towels at Supply Side Kitchen, where n is the number of cases of papertowels.Paper Towels(cases)Restaurant WarehouseSupply Side5$262.20$202.2010$427.15$382.1515$592.10$562.10r(n) =n+s(n) =n +forcases ofBoth Restaurant Warehouse and Supply Side charge $paper towels.

Answer 1

The slope-intercept form of a line is:

y = mx + b

where m is the slope and b is the y-intercept

The slope of the line that passes through the points (x1, y1) and (x2, y2) is computed as follows:

`m=(y_2-y_1)/(x_2-x_1)`

Restaurant warehouse's line passes through (5, 262.2) and (10, 427.15). Its slope is:

`m=(427.15-262.2)/(10-5)=(164.95)/(5)=32.99`

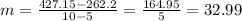
Supply side's line passes through (5, 202.2) and (10, 382.15). Its slope is:

`m=(382.15-202.2)/(10-5)=(179.95)/(5)=35.99`

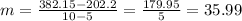
Replacing with the point (5, 262.2) and m = 32.99 in the general equation:

262.2 = 32.99(5) + b

262.2 - 164.95 = b

97.25 = b

Replacing with the point (5, 202.2) and m = 35.99 in the general equation:

202.2 = 35.99(5) + b

202.2 - 179.95 = b

22.25 = b

Then, the equations are:

r(n) = 32.99n + 97.25

s(n) = 35.99n + 22.25

If both restaurants charge the same, then r(n) = s(n)

32.99n + 97.25 = 35.99n + 22.25

97.25 - 22.25 = 35.99n - 32.99n

75 = 3n

75/3 = n

25 = n

r(25) = 32.99(25) + 97.25 = 922

Both restaurants charge $922 for 25 cases of paper towels