The Solution:
Given the graph in the picture on the Question section.
We are required to show how to find the slope of a line and interpret it.
Step 1:
The formula for finding the slope of the line is:
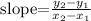
Step 2:
Pick two coordinates on the line.
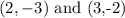
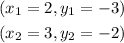
Step 3:
Substituting the above values in the formula for slope, we get
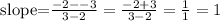
The slope is a positive slope, which means that when the value of x is increasing then the value of y is increasing. Also, for every unit change in the value of y, there is a corresponding unit change in the value of x.
Therefore, the slope is 1.