Question

Hi, can you help me answer this question, please, thank you

Answer 1

Given the information on the problem, we have that the population is normally distributed, then, the test statistic is the following:

`Z=\frac{\bar{X}-\mu}{\frac{\sigma}{\sqrt[]{n}}}`

in this case we have:

`\begin{gathered} \bar{X}=58 \\ \mu=81.2 \\ \sigma=17.9 \\ n=11 \\ \Rightarrow Z=\frac{58-81.2}{\frac{17.9}{\sqrt[]{11}}}=(-23.2)/(5.397)=-4.298 \\ Z=-4.298 \end{gathered}`

then, the test statistic is Z = -4.298.

For the p-value, notice that since we want to test the hypothesis H1: mu < 81.2, the p-value of the test is the probability that a random sample of size 11 will have a mean of 58 or les if the real mean would be 81.2, this is:

`P(Z\leq-4.298)=0`

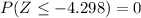
therefore, the p-value is 0