Question

I really need help with this practice problem It asks to answer (a) and (b)

Answer 1

We want to get the value of r from the ratio test from the series;

`\sum ^(\infty)_(n\mathop=1)((2n!)/(2^(2n)))`

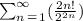
The ratio test is;

`\lim _(n\to\infty)\lvert(a_(n+1))/(a_n)\rvert`

We can find the limit as;

`\begin{gathered} a_n=(2n!)/(2^(2n)) \\ a_(n+1)=(2(n+1)!)/(2^(2n+1)) \end{gathered}`

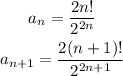
The quotient is; '

`(a_(n+1))/(a_n)=\frac{2(n+1)!(2^(2n))_{}}{2^(2(n+1))(2n!)}=(2(n+1)* n!*2^(2n))/(2*2^2*2^(2n)* n!)=(n+1)/(4)`

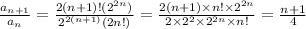
Therefore;

`r=(n+1)/(4)`

b. What does this value of r tell you about the series;

Taking the limit of the ratio r;

`\lim _(n\to\infty)((n+1)/(4))=\infty_{}`

We can tell that the series is divergent.