Question

A projectile is launched upward from ground level with an intentional speed of 98 m/s. How high will it go? when will it return to the ground?

Answer 1

check the picture below.

now, keeping in mind that gravity will pull the projectile down, and that'd be 9.8 m/s², that gets halfed for the initial velocity equation, so 9.8/2 or 4.9 m/s².


`\bf ~~~~~~\textit{initial velocity}\\\\ \begin{array}{llll} ~~~~~~\textit{in meters}\\\\ h(t) = -4.9t^2+v_ot+h_o \end{array} \quad \begin{cases} v_o=\stackrel{98}{\textit{initial velocity of the object}}\\\\ h_o=\stackrel{\textit{from the ground thus }0}{\textit{initial height of the object}}\\\\ h=\stackrel{}{\textit{height of the object at`

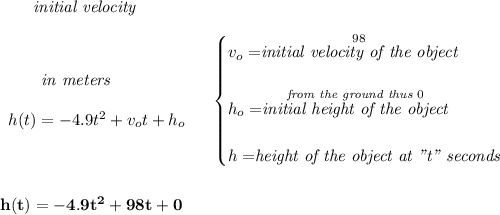
how high will it go? well, notice in the picture, it'll go as high as its vertex,


`\bf \textit{ vertex of a vertical parabola, using coefficients}\\\\ \begin{array}{lccclll} h(t) = &{{ -4.9}}t^2&{{ +98}}t&{{ +0}}\\ &\uparrow &\uparrow &\uparrow \\ &a&b&c \end{array}\qquad \left(-\cfrac{{{ b}}}{2{{ a}}}\quad ,\quad {{ c}}-\cfrac{{{ b}}^2}{4{{ a}}}\right) \\\\\\ \left(\stackrel{\textit{how long it took}}{-\cfrac{98^2}{2(-4.9)}}~~,~~\stackrel{\textit{how high it went}}{0-\cfrac{98^2}{4(-4.9)}} \right) \\\\\\ 0-\cfrac{9604}{-19.6}\implies 490`

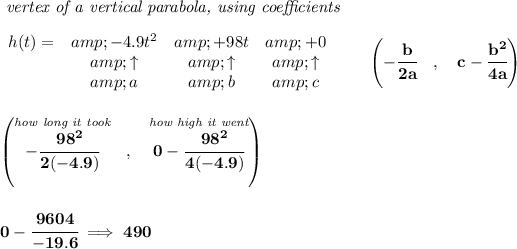
when will it return to the ground? well, notice the picture, when y = 0.


`\bf \stackrel{h(t)}{0}=-4.9t^2+98t+0\implies 4.9t^2-98t=0\implies t(4.9t-98)=0\\\\ -------------------------------\\\\ t=0\\\\ -------------------------------\\\\ 4.9t-98=0\implies 4.9t=98\implies t=\cfrac{98}{4.9}`

so after "t" seconds it comes back down.

notice is 0 at two points, at t=0 or 0 seconds, because it just took off the ground, and again the second time, when it comes back down.