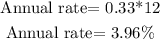Answer:

Explanation:
It is given that the value of a necklace increases by 3.2% per year, then by the equation of future value, the value of the necklace after t years will be:
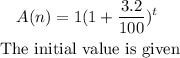
Now, since the necklace after 1 year will be $1.032. From the following equation determine the value of the ring after 1 year:

Therefore, the ring will value more after 1 year. This is because the ring has a greater annual rate than the necklace.