Question

Find the area of the shaded portion of the figures below . Use straight pi= 3.

Answer 1

The area of a circle is given by the following formula:

`A_C=\pi\cdot r^2`

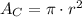
where r is the lenght of the circle's radius.

In this case, we have a radius of 12 units, so the area will be

`A_C=\pi\cdot12^2=3.14\cdot144=452.16`

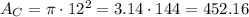
We are however, asked to find the area of the shaded region, which is encased within a square. From the image we can see that the circle's radius is half the length of a side of the square. In other words, the square's sides measure 24 units.

The area of a square is given by

`A_S=s^2`

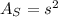
where s is the lenght of the sides of the square. In this case,

`A_S=24^2=576`

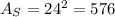
Now, in order to determine the area of the shaded region, we subtract the area of the circle from the area of the square:

`A_R=A_S-A_C=576-452.16=123.84`

So the area of the shaded region is 123.84 square units.