Question

Simplfy the Radical 18) 9*sqrt(125)

Answer 1

Given the expression:

`9\cdot\sqrt[]{125}`

notice that for 125 we have the following:

`125=5^3`

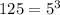
therefore, we can simplify the expression by using 5^3 to get the following:

`9\cdot\sqrt[]{125}=9\cdot\sqrt[]{5^3}=9\cdot\sqrt[]{5^2\cdot5}`

now, remember that when we have a product inside a square root, we can split it on both factors. In general, for any exponent, we have the following rule:

`\begin{gathered} (a\cdot b)^n=a^nb^n \\ \text{ in this case:} \\ \sqrt[]{a\cdot b}=\sqrt[]{a}\cdot\sqrt[]{b} \end{gathered}`

then, for our expression at hand, we have:

`9\cdot\sqrt[]{5^2\cdot5}=9\cdot\sqrt[]{5^2}\cdot\sqrt[]{5}=9\cdot5\cdot\sqrt[]{5}=45\sqrt[]{5}`

therefore, the simplifed expression is 45*sqrt(5)