Question

Find the absolute extrema on the function of the given interval

Answer 1

Notice that:

`\begin{gathered} 6x^2-24x=6(x^2-4x), \\ 6x^2-24x=6\mleft(x^2-4x+4-4\mright), \\ 6x^2-24x=6((x-2)^2-4), \\ 6x^2-24x=6(x-2)^2-24. \end{gathered}`

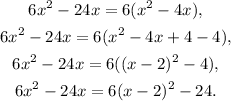
Therefore, f(x) has an absolute minimum of -24 at x=2.

Since the given function is a parabola (in the interval [0,5]) with a leading coefficient greater than zero and vertex at (2,-24) we get that it has an absolute maximum at x=5, which is:

`\begin{gathered} f(5)=6\cdot5^2-24\cdot5, \\ f(5)=6\cdot25-120, \\ f(5)=150-120, \\ f(5)=30. \end{gathered}`

Answer:

Absolute maximum=(5, 30).

Absolute minimum=(2, -24).