Question

compare the x-intercepts for the two linear functions represented in the table below. which of the following statements is true?

Answer 1

• Given Function A:

`-4x+2y=-2`

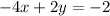
You need to remember that, by definition, the value of "y" is zero when the function intersects the x-axis.

Therefore, you need to set up that:

`y=0`

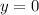
Substitute this value into the function and then solve for "x", in order to find the x-intercept:

`\begin{gathered} -4x+2(0)=-2 \\ -4x=-2 \\ \\ x=(-2)/(-4) \\ \\ x=(1)/(2) \end{gathered}`

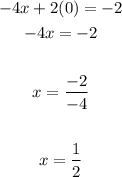
• Given that Function B passes through these points:

`(0,-1),(3,2)`

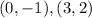
You need to determine the equation of the line, in order to find the x-intercept using it.

The Slope-Intercept Form of the equation of a line is:

`y=mx+b`

Where "m" is the slope of the line and "b" is the y-intercept.

By definition, the value of "x" is zero when the function intersects the y-axis. Therefore, knowing the first point given in the exercise (whose x-coordinate is zero), you can determine that, for this line:

`b=-1`

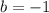
Substitute "b" and the coordinates of the second point into this equation:

`y=mx+b`

And then solve for "m":

`\begin{gathered} 2=m(3)-1 \\ 2+1=3m \\ \\ (3)/(3)=m \\ \\ m=1 \end{gathered}`

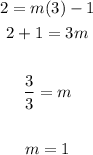
Therefore, the equation of Function B in Slope-Intercept Form is:

`y=x-1`

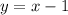
Now you can find the x-intercept by using the procedure used for Function A:

`\begin{gathered} 0=x-1 \\ x=1 \end{gathered}`

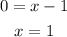
You know that:

`(1)/(2)<1`

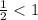
Hence, the answer is: Third option.