Question

Instructions: For the following quadratic functions, write the function in factored form and then find the -intercepts, axis of symmetry, vertex, and domain and range.

Answer 1

Given the function:

`y=x²-2x-8`

we have that the factored form is:

`y=(x-4)(x+2)`

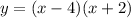
with this representation, we can see that the x-intercepts are:

`\begin{gathered} x=4 \\ x=-2 \end{gathered}`

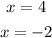
Next, the axis of symmetry can be found with the following expression:

`x=-(b)/(2a)`

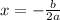
in this case, a = 1 and b = -2 (since a and b are the main coefficients on the equation), then, the axis of symmetry is:

`x=-(-(2))/(2(1))=1\Rightarrow x=1`

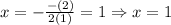
The vertex can be found by evaluating the axis of symmetry on the equation. then, if we make x = 1, we get:

`y=(1)²-2(1)-8=1-2-8=-9`

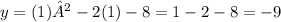
therefore, the vertex is the point (1,-9).

Finally, the domain of the function is the set of all real numbers (-inf,inf), since it is a polynomial function. The range is [-9,inf), since the vertex is located at the point (1,-9)