Question

A recent poll of 80 randomly selected Californians showed that 38% ( = 0.38) believe they are doing all that they can to conserve water.

The state government would like to know, within a 99% confidence level, the margin of error for this poll. (99% confidence level z*-score of 2.58)

Remember, the margin of error, E, can be determined using the formula E = z*.

To the nearest whole percent, the margin of error for the poll is %.

Answer 1

Answer: 14%

Explanation:

We know that Margin error
`M.E.=z\sqrt{(p(1-p))/(n)}`

Given: Sample size n=80

sample proportion p=0.38

Confidence level z=2.58

Substitute the values in the formula, we get

Margin error
`M.E.=2.58\sqrt{(0.38(1-0.38))/(80)}`

`\Rightarrow\ M.E.=2.58\sqrt{(0.62\ \cdot\ 0.38)/(80)}\\\Rightarrow\ M.E.=2.58 √(0.002945)\\\Rightarrow\ M.E.=2.58*0.054\\\Rightarrow\ M.E.=0.13932\\\Rightarrow\ M.E.=13.932\%\approx14\%`

Thus, to the nearest whole percent, the margin of error for the poll is 14%

Answer 2

The margin of error given the proportion can be found using the formula


`z* \sqrt{ (p(1-p))/(n) }`

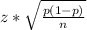
Where

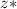
`z*` is the z-score of the confidence level

`p` is the sample proportion

`n` is the sample size

We have

`z*=2.58`

`p=0.38`

`n=80`

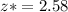
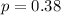
Plugging these values into the formula, we have:

`2.58 \sqrt{ (0.38(1-0.38))/(80) } =0.14`

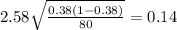
The result 0.14 as percentage is 14%

Margin error is 38% ⁺/₋ 14%