Question

3. To produce x units of a religious medal costs C(x) =12x + 29. The revenue is R(x) = 25x. Both C(x) and R(x) are in dollars.a. Find the break-even quantity (x value)b. Find the profit from 250 units.c. Find the number of units that must be produced for a profit of $130.

Answer 1

Part a.

The break-even point is where the cost equals to the revenue, that is,

`C(x)=R(x)`

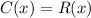
Then, by substituting the given information, we get

`12x+29=25x`

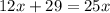
So, by subtracting 12x to both sides, it yields,

`\begin{gathered} 29=13x \\ or\text{ equivalently, } \\ 13x=29 \end{gathered}`

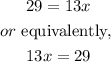
Then, x is given by

`x=(29)/(13)=2.2307`

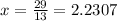
Then, by rounding to the nearest whole number, the break even quantity is 2 medals.

Part b.

The profit is equal to the revenue minus the cost, that is,

`P(x)=R(x)-C(x)`

So we have

`P(x)=25x-(12x+29)`

which gives

`P(x)=13x-29`

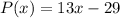
By substituting x=25o into this result ,we have

`\begin{gathered} P(250)=13(250)-29 \\ P(250)=3250-29 \\ P(250)=3221 \end{gathered}`

Therefore, the profit from 250 units is $3221.

Part c

In this case, we need to substitute P=130 into the profit function and find x, that is,

`130=13x-29`

So, by adding 29 to both sides, we have

`\begin{gathered} 159=13x \\ or\text{ equivalently, } \\ 13x=159 \end{gathered}`

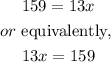
Therefore, we have

`\begin{gathered} x=(159)/(13) \\ x=12.23 \end{gathered}`

Therefore, by rounding to the nearest whole number, the number of medals to produce a profit of $130 is 12 medals.