Question

Find the length of the shorter legs of a right triangle if the longer leg is 24 m and the hypotenuse is six more than twice the shorter leg

Answer 1

the length of the shorter leg = 10m

Step-by-step explanation:

longer leg = 24m

shorter leg = ?

hypotenuse = 6 more than twice the shorter leg

`\text{hypotenuse = 6 + 2(shorter leg)}`

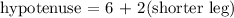
Since the triangle is a right angled triangle, to get the shorter leg we will apply pythagoras theorem:

Hypotenuse² = opposite² + adjacent²

Hypotenuse² = (shorter leg)² + (longer leg)²

substitute the values in the formula:

`\begin{gathered} \lbrack6+2(shorter\text{ }leg)\rbrack^2\text{ = (shorter leg)}^2+(\text{24})^2 \\ \text{let x represent shorter leg} \\ \lbrack6+2(x)\rbrack^2\text{ = (x)}^2+(\text{24})^2 \\ \lbrack6+2x\rbrack^2\text{ = x}^2+24^2 \end{gathered}`
`\begin{gathered} (6+2x\rbrack)(6+2x)\text{ = x}^2+576 \\ 6(6\text{ + 2x) + 2x(6 + 2x) = x}^2+576 \\ 36+12x+12x+4x^2\text{ = x}^2+576 \\ \text{collect like terms:} \\ 4x^2\text{- x}^2+36+12x+12x=576 \end{gathered}`
`\begin{gathered} 3x^2+36+24x=576 \\ 3x^2+24x+36-576\text{ = 0} \\ 3x^2+24x-540\text{ = 0} \\ \text{divide through by 3:} \\ x^2+8x-180\text{ = 0} \end{gathered}`

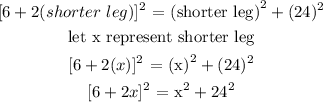
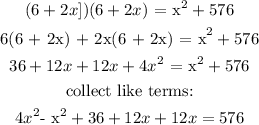
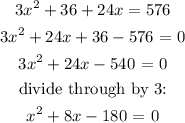
To get x, we will apply factorisation method:

`\begin{gathered} x^2\text{ + 18x - 10x - 180 = 0} \\ x(x\text{ + 18) -10(x + 18) = 0} \\ (x\text{ - 10)(x + 18) = 0} \\ x\text{ - 10 = 0 or x + 18 = 0} \\ x\text{ = 10 or x = -18} \end{gathered}`

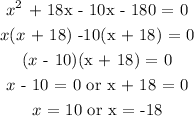
Since we can't have a negative length, the value of x will be 10

As a result, the length of the shorter leg = 10m