Question

Find sum of geometric series where a1= 125, r=2/5, n=4

Answer 1

First, we must remember that if a sequence is geometric, that of finding the sum of the first n terms, called Sn, without having to add all the terms.

To do this, we use the following equation:

`S_n=(a_1(1-r^n))/(1-r)`

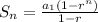
Where in this case we have:

`\begin{gathered} a_1=125 \\ r=(2)/(5) \\ n=4 \end{gathered}`

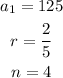
Now, we replace these values and solve the sum:

`\begin{gathered} S_4=125\cdot((1-((2)/(5))^4))/((1-(2)/(5))) \\ S_4=125\cdot((1-(16)/(25)))/((1-(2)/(5))) \\ S_4=125\cdot((609)/(625))/((3)/(5)) \\ S_4=125\cdot(609\cdot5)/(625\cdot3) \\ S_4=125\cdot(203)/(125) \\ S_4=203 \end{gathered}`

In conclusion, the sum of the geometric series with a1= 125, r=2/5, n=4​ is a total of 203.