Question

Solve the following system of equations by using the elimination method.x - y = 112x + y = 19

Answer 1

`\begin{gathered} x-y=11 \\ 2x+y=19 \end{gathered}`

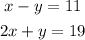
To solve a system of equations by elimination method you need to have one f the variables ( x or y) with opposite coefficient in the two equations of the system.

In this case you have the variable y with opposite coefficients; -1 and +1

You add the equations as follow:

As you get that the sum of the equations is:

`3x=30`

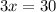
You solve the x:

- Divide both sides of the equation into 3:

`\begin{gathered} (3)/(3)x=(30)/(3) \\ \\ x=10 \end{gathered}`

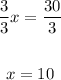
You use this value of x to find the value of y by substitute the x in one of the equation by 10:

`10-y=11`

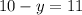
Solve for y:

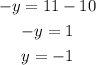
`\begin{gathered} -y=11-10 \\ -y=1 \\ y=-1 \end{gathered}`Then the solution for the system of equations is: x= 10 and y= -1