Question

Find the surface area of the regular pyramid shown in the accompanying diagram. If necessary, express your answer in simplest radical form.The pyramid has a square base, an edge length of 10 units and a height of 12 units.A. 260 units²B. 360 units²C. 340 units²D. 300 units²

Answer 1

Given that

There is a square pyramid with its height, h = 12 units and base edge, a = 10 units

And we have to find the surface area of the pyramid.

Explanation -

The surface area of the square pyramid is given as

`\begin{gathered} Area=a^2+2a\sqrt{(a^2)/(4)+h^2} \\ \\ where\text{ a = base edge length and h = height} \end{gathered}`

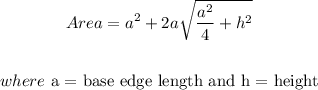
On substituting the values we have

`\begin{gathered} A=10^2+2*10\sqrt{(10^2)/(4)+12^2} \\ \\ A=100+20\sqrt{(100)/(4)+144} \\ \\ A=100+20√(25+144) \\ \\ A=100+20√(169) \\ \\ A=100+20√(13*13) \\ A=100+20*13\text{ sq units} \\ A=100+260\text{ sq units} \\ A=360\text{ sq units} \end{gathered}`

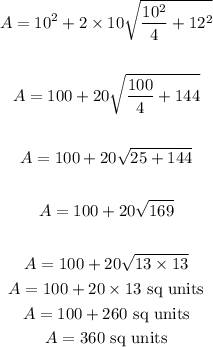
So the required area is 360 sq units and OPTION B is correct.

Final answer -

Therefore the final answer is 360.