Question

Hi I’m looking to get a step by step solution in solving this problem preparing for my test

Answer 1

To find the solution of the equation we need to remember that the logarithm functions and the exponential functions are inverse of each other that is:

`\log_bb^x=x`

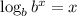
Then we apply the correct logarithm to both sides of the equation to get:

`\begin{gathered} 3^x=17 \\ \log_33^x=\log_317 \\ x=\log_317 \end{gathered}`

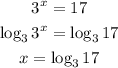
Now, if we want to write the solution in terms of the natural logarithm, we need to remember that:

`\log_bx=(\ln x)/(\ln b)`

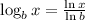
Therefore, the solution of the equation can be express in the two following ways:

`\begin{gathered} x=\log_317 \\ \text{ or} \\ x=(\ln17)/(\ln3) \end{gathered}`