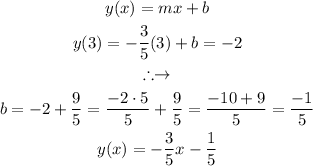Question

Find the equation of the line passing through the points (3,-2) and (-2, 1) write the equation in slope intercept and standard form

Answer 1

`y(x)=-(3)/(5)x-(1)/(5)`

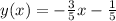
Step-by-step explanation: We need to find the equation of the line in y-intercept form:

Given the two points:

`\begin{gathered} P_1(3,-2) \\ P_2(-2,1) \end{gathered}`

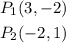
The standard form of the equation of the line is:

`y(x)=mx+b`

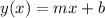
Where:

`\begin{gathered} m=(\Delta y)/(\Delta x)\rightarrow slope \\ b\rightarrow y-intercept \end{gathered}`

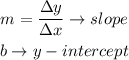
Now, the last step is to find these unknowns from the given information:

Slope:

`m=(\Delta y)/(\Delta x)=(1-(-2))/(-2-(3))=(1+2)/(-2-3)=(3)/(-5)=-(3)/(5)`

Y-intercept:

We will simply now put one of the points in our standard equation, and extract the y-intercept:

`\begin{gathered} y(x)=mx+b \\ y(3)=-(3)/(5)(3)+b=-2 \\ \therefore\rightarrow \\ b=-2+(9)/(5)=(-2\cdot5)/(5)+(9)/(5)=(-10+9)/(5)=(-1)/(5) \\ y(x)=-(3)/(5)x-(1)/(5) \end{gathered}`