Question

Identify the binomial that is a factor of the polynomial.

Answer 1

Given:

`f(x)=2x^3+12x^2-26x-84`

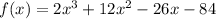
To find the binomial that is a factor of the polynomial:

By factor theorem,

If f(a) = 0, then the binomial (x - a) is a factor of polynomial f(x).

So, let us check the options one by one.

A) Put x = -7 in the given polynomial.

`\begin{gathered} f(-7)=2(-7)^3+12(-7)^2-26(-7)-84 \\ =2(-343)+12(49)+182-84 \\ =0 \end{gathered}`

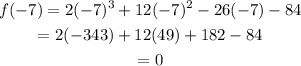
Since, f(-7)=0

Therefore, (x+7) is a factor.

Thus, the correct option is A.