Question

For which of the following intervals does the function (insert image) have a removable discontinuity?A. [−2.5,−1.5] B. [−1.5,−0.5] C. [−0.5,0.5] D. [0.5,1.5] E. [1.5,2.5]

Answer 1

`f(x)=(x^2-4x+3)/((x^2+x-2)(x^2-2x-3))`

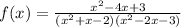
First, we need to factorize the quadratic expressions. To do this we need to find the roots of each quadratic expression. In the function of the numerator, the coefficients are: a = 1, b = -4, and c = 3. Applying the quadratic formula, the roots are:

`\begin{gathered} x_(1,2)=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ x_(1,2)=\frac{4\pm\sqrt[]{(-4)^2-4\cdot1\cdot3}}{2\cdot1} \\ x_(1,2)=\frac{4\pm\sqrt[]{4}}{2} \\ x_1=(4+2)/(2)=3 \\ x_2=(4-2)/(2)=1 \end{gathered}`

Then, we can express the function with its roots as follows:

`\begin{gathered} ax^2+bx+c=a(x-x_1)(x-x_2) \\ x^2-4x+3=(x-3)(x-1) \end{gathered}`

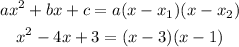
In the first function of the denominator, the coefficients are: a = 1, b = 1, and c = -2. Applying the quadratic formula, the roots are:

`\begin{gathered} x_(1,2)=\frac{-1\pm\sqrt[]{1^2-4\cdot1\cdot(-2)}}{2\cdot1} \\ x_(1,2)=\frac{-1\pm\sqrt[]{9}}{2} \\ x_1=(-1+3)/(2)=1 \\ x_2=(-1-3)/(2)=-2 \end{gathered}`

Then, we can express the function with its roots as follows:

`x^2+x-2=(x-1)(x+2)`

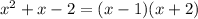
In the second function of the denominator, the coefficients are: a = 1, b = -2, and c = -3. Applying the quadratic formula, the roots are:

`\begin{gathered} x_(1,2)=\frac{2\pm\sqrt[]{(-2)^2-4\cdot1\cdot(-3)}}{2\cdot1} \\ x_(1,2)=\frac{2\pm\sqrt[]{16}}{2} \\ x_1=(2+4)/(2)=3 \\ x_2=(2-4)/(2)=-1 \end{gathered}`

Then, we can express the function with its roots as follows:

`x^2-2x-3=(x-3)(x+1)`

Substituting the equivalent expression into the original rational function, we get:

`\begin{gathered} f(x)=((x-3)(x-1))/((x-1)(x+2)(x-3)(x+1)) \\ \text{ Simplifying:} \\ f(x)=(1)/((x+2)(x+1)) \end{gathered}`

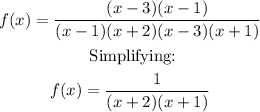
After the simplification, the discontinuities at x = 3 and x = 1 have been removed. x = 1 belongs to the interval [0.5, 1.5]