Question

Iron reacts with hydrochloric acid to produce iron (II) chloride and hydrogen gas: Fe(s) + 2 HCl(aq) n FeCl2(aq) + H2(g) The H2 gas from the reaction of 2.2 g of iron with excess acid is collected in a 10.0-L flask at 25 °C. What is the pressure of the H2 gas in this flask?

Answer 1

0.095 atm.

Step-by-step explanation:

What is given?

Mass of iron (Fe) = 2.2 g.

Molar mass of Fe = 56 g/mol.

Volume (V) = 10.0 L.

Temperature (T) = 25°C = 25° C + 273 = 298 K.

What do we need? The pressure of H2.

Step-by-solution:

First, we need to find the number of moles of H2 that we obtain from 2.2 g of Fe. Let's find the number of moles of Fe that are in 2.2 g using its molar mass:

`2.2\text{ g Fe}\cdot\frac{1\text{ mol Fe}}{56\text{ g Fe}}=0.039\text{ moles Fe.}`

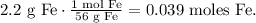
You can see in the chemical equation that 1 mol of Fe produces 1 mol of H2, so the molar ratio between these two compounds is 1:1. This means that 0.039 moles of Fe reacted will produce 0.039 moles of H2 too, so the number of moles of H2 is 0.039:

`0.039\text{ moles Fe}\cdot\frac{1\text{ mol H}_2}{1\text{ mol Fe}}=0.039\text{ moles H}_2.`

Now, we want to know what is the pressure of the gas in the flask, so based on the data that we have, we use the formula of ideal gas law, which is:

`PV=nRT.`

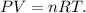
Where P is pressure, V is volume, n is the number of moles, R is the constant of ideal gas which, in this case, is 0.082 atm*L/mol*K, and T is temperature. Let's solve for P and replace the given data in the new formula:

`P=(nRT)/(V)=\frac{0.039\text{ moles}\cdot0.082(atm\cdot L)/(mol\cdot K)\cdot298K}{10.0\text{ L}}=0.095\text{ atm.}`

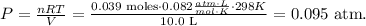
The pressure of the H2 gas in the flask is 0.095 atm.