Question

A ramp 18 ft long rises to a platform that is 16 ft off the ground. Find x, the angle of elevation of the ramp. Round youranswer to the nearest tent of a degree.

Answer 1

The triangle is a right angle triangle with the following parameters given in the diagram

`\begin{gathered} \text{angle of elevation,}\theta=x \\ \text{Hypotenuses =18ft} \\ \text{opposite side=16ft} \end{gathered}`

Using the trigonometry ratio for the sine of an angle, we have

`\sin \theta=(opposite)/(hypotenuse)`

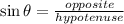
Then we substituting the parameters into the formula above, we obtain

`\begin{gathered} \sin x=(16)/(18) \\ \sin x=(8)/(9) \\ \sin x=0.8889 \end{gathered}`

Then the value of x becomes

`\begin{gathered} x=\sin ^(-1)(0.8889) \\ x=62.7^0 \end{gathered}`

Therefore x= 62.7°