Given the measures for the diameters of ball beraings
16.19, 16.21, 16.26, 16.36, 16.38, 16.42, 16.45
You have to calculate the mean and standard deviation.
1) To calculate the mean you have to use the following formula:
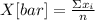
2) To calculate the standard deviation you have to calculate the variance first and the the square root of the variance using the formulas:
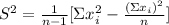
![S=\sqrt[]{S^2}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/5p00vuslhtnzqxbhgf0e.png)
As you see both formulas involve the summation of the observations and the summation of the squared observations, so those are the first calculations you have to do:
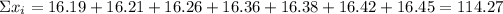
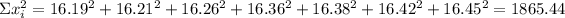
There are 7 oobservations in the data set so n=7
1) The mean is
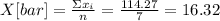
2) The variance is
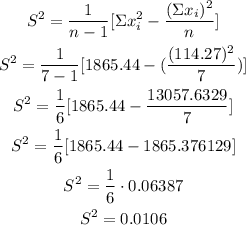
Now to determine the standard deviation you have to calculate the square root of the variance:
![\begin{gathered} S=\sqrt[]{S^2} \\ S=\sqrt[]{0.0106} \\ S=0.103\cong0.1 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/9f2omi8j4j2ijna25dbr.png)
The meand is X[bar]=16.32mm and the standard deviation is S=0.1 mm