Question

A bond has a face amount of $10,000 and matures in 10years. The nominal rate of interest on the bond is 6.4%. Atwhat price would the bond yield a true rate of interest of6.5%? 1) Calculate the semi-annual interest payments 2)Calculate the present value of the payments 3) Calculate thepresent value of the bond at maturity 4) Find the price ofthis bond 5) Explain the adjusted current yield and currentyield.

Answer 1

Given:

Face value, P = $10000

Time, t = 10 years

Rate, R = 6.4% = 0.064

Let's solve for the following:

• 1) Calculate the semi-annual interest payments.

Apply the formula:

`S=(P\ast R)/(2)`

Thus, we have:

`\begin{gathered} S=(10000\ast0.064)/(2) \\ \\ S=(640)/(2) \\ \\ S=320 \end{gathered}`

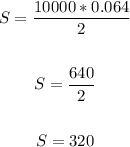
The semi-annual interest payment is $320

• (2) Calculate the present value of the payments.

To find the present value, apply the formula:

`PV=(PMT)/(r)\ast\lbrack1-(1)/((1+r)^n)\rbrack`

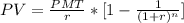
Where:

r = 0.065/2 = 0.0325

n is the number of periods = 10 x 2 = 20

PMT = 320

Thus, we have:

`\begin{gathered} PV=(320)/(0.0325)\ast\lbrack1-(1)/((1+0.0325)^(20))\rbrack \\ \\ PV=9846.15\ast\lbrack1-0.52747\rbrack \\ \\ PV=9846.15\ast0.472529 \\ \\ PV=4652.59 \end{gathered}`

The present value of the payments is $4652.59

3) Calculate the present value of the bond at maturity.

Take the formula:

`PV=\frac{face\text{ value}}{(1+r)^n}`

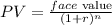
Thus, we have:

`\begin{gathered} PV=(10000)/((1+0.0325)^(20)) \\ \\ PV=(10000)/(1.8958) \\ \\ PV=5274.71 \end{gathered}`

The present value of the bonds at maturity is $5274.71

• 4) Find the price of this bond.

To find the price f this bond, we have:

Price of bond = Present value of payments + Present value at maturity

Price of bond = $4652.59 + $5274.71

Price of bond = $9927.30

The price of this bond is $9,927.30

5) To find the current yield, apply the formula:

`C=\frac{annual\text{ payment}}{\text{current price of bond }}\ast100`

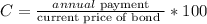
Thus, we have:

`\begin{gathered} (320\ast2)/(9927.30)\ast100 \\ \\ =(640)/(9927.30)\ast100 \\ \\ =0.0645\ast100 \\ \\ =6.45\text{ \%} \end{gathered}`

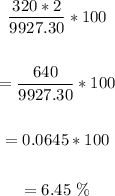
The current yield is 6.45%

ANSWER:

1) $320

2) $4,652.59

3) $5,274.71

4) $9,927.30

5) 6.45%