Question

The function V (t) = 1.50(1.17)^t represents the value V (t), in dollars, of a comic book t years after its purchase in 2000. Determine the annual growth rate in the value of the comic book and its value in 2025. The comic book will be worth $ _____ in the year 2025The annual growth rate of the comic book is ______%The answer box1.50 1.17 17 117

Answer 1

The function for the value is:

`V(t)=1.50\cdot(1.17)^t`

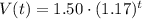
The annual growth rate is the increase in value for a year t to a year t+1, so we can find this rate by calculating the quotient [V(t+1)-V(t)] / V(t).

[V(t+1)-V(t)] represents the increase in value as the difference between the value in each year, and V(t) makes it to be relative to the year of reference.

`\begin{gathered} (V(t+1)-V(t))/(V(t))=(V(t+1))/(V(t))-(V\mleft(t\mright))/(V(t))=(V(t+1))/(V(t))-1 \\ \\ (V(t+1))/(V(t))-1=(1.50\cdot1.17^(t+1))/(1.50\cdot1.17^t)-1=1.17^(t+1-t)-1=1.17^1-1=0.17 \end{gathered}`

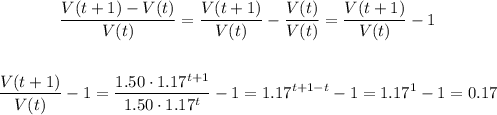
The annual growth rate is 0.17 or 17%.

The year 2025 is 25 years from 2000 so the value of t is t=2025-2000=25.

We can then calculate the value for V(25) as:

`\begin{gathered} V(25)=1.50\cdot1.17^(25)\approx1.50\cdot50.66 \\ V(25)\approx75.99 \end{gathered}`

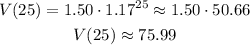
Answer:

The comic book will be worth $ 75.99 in the year 2025.

The annual growth rate of the comic book is 17%.