Question

Line Equation from Two PointsSep 22,5/35:32 PMWatch help videoWhat is an equation of the line that passes through the points (-7, -6) and(-1,0)AnswerSubww Answer

Answer 1

We must find the equation of the line that passes through points A = (-7,-6) and B = (-1,0).

The general slope-intercept equation of a line is:

`y=m\cdot x+b,`

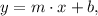
where the b is the y-intercept and m is the slope of the line.

1) We compute the slope using the following formula:

`m=\frac{y_B-y_A}{x_B-x_A_{}}_{}_{},`

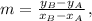
where (xA,yA) and (xB,yB) are the coordinates of the points A and B, respectively.

Replacing the coordinates of the points of the problem, we find that:

`m=(0-(-6))/(-1-(-7))=1.`

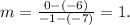
2) To compute the y-intercept b, we replace the value of m = 1 and the coordinates of one of the points (B for example) in the general equation of the line, and then we solve for b:

`\begin{gathered} y_B=m\cdot x_B+b, \\ 0=1\cdot(-1)+b, \\ b=1. \end{gathered}`

Replacing the values m = 1 and b = 1 in the equation of the line, we find that:

`y=x+1`

Answer

The equation of the line is:

`y=x+1`