Question

How many items does the company need to sell in order to be profitable?

Answer 1

EXPLANATION

Let's see the facts:

Revenue --> R(x) = 2sqrt (x) - 4 [x=number of items sold]

Cost --------> C(x) = 6 - sqrt (x)

The company will be profitable when the Revenue exceeds the cost.

Profitable condition: Revenue > Cost

Setting the two functions equal to each other and solving for x:

`2\sqrt[]{x}-4\text{ = 6 - }\sqrt[]{x}`

Adding +sqrt(x) to both sides:

`2\sqrt[]{x}+\sqrt[]{x}-4\text{ = 6 }`

Adding +4 to both sides:

`2\sqrt[]{x}+\sqrt[]{x}\text{ = 6 }+4`

Adding similar terms and simplifying:

`3\sqrt[]{x}=10`

Dividing both sides by 3:

`\sqrt[]{x}=10/3`

Applying the power of 2 to both sides:

`x=(10/3)^2`

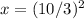
Simplifying:

x=11.11

The answer is about 111. In order for a company to be profitable, their revenue must exceed their cost. Setting the two functions equal to each other and solving for x gives the minimum number of items that need to be sold in order to be profitable. OPTION B.