Question

Determine whether the given line has a positive slope, a negative slope, zero slope, or an undefined slope.A.negative slopeB.postitive slopec. zero slopeD.Undefined slope

Answer 1

The graph of the constant function y = c is a horizontal line in the plane that passes through the point (0, c).

In the context of a polynomial in one variable x, the non-zero constant function is a polynomial of degree 0 and its general form is f(x) = c where c is nonzero. This function has no intersection point with the x-axis, that is, it has no root (zero). On the other hand, the polynomial f(x) = 0 is the identically zero function. It is the (trivial) constant function and every x is a root. Its graph is the x-axis in the plane.

The graph below is a constant graph function at

`\begin{gathered} c=3 \\ \text{this is a graph of } \\ y=3 \end{gathered}`

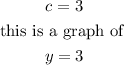
This means that the y-intercept is

`=(0,3)`

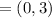
The general equation of a line is given as

`\begin{gathered} y=mx+c \\ \text{where} \\ m=\text{slope} \\ c=\text{intercept} \end{gathered}`

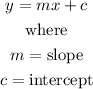
Since its a constant graph, that means the graph has the repeated domain for the same value of the range at y =2

Therefore,

the coordinates of the line will be

`\begin{gathered} (0,3)\text{ and (2,3)} \\ x_1=0 \\ y_1=3 \\ x_2=2 \\ y_2=3 \end{gathered}`

The formula us to calculate the slope of the line is given below as

`\begin{gathered} m=(y_2-y_1)/(x_2-x_1) \\ \text{substitiuting the values, we will have} \\ m=(3-3)/(2-0) \\ m=(0)/(2) \\ m=0 \end{gathered}`

Therefore,

The line in the graph has a ZERO SLOPE

The Final answer is OPTION C