Question

The difference of two whole numbers is 2. If two times the square of the smaller number added to three times the square of the larger number equals 140, find the numbers.

Answer 1

Statement 1:

Let the smaller number be x

Let the larger number be y

The difference between two whole numbers is 2 can be written as

`y-x=2`

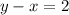
Statement 2:

Two times the square of the smaller number is:

`2* x^2=2x^2`

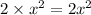
Three times the square of the larger number is:

`3* y^2=3y^2`

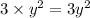
Thus,

`2x^2+3y^2=140`

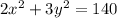
Therefore we would solve the system of equations to find the two numbers

`\begin{gathered} From\text{ statement 1} \\ y=2+x \\ \therefore \\ 2x^2+3(2+x)^2=140 \\ 2x^2+3((x+2)(x+2))=140 \end{gathered}`
`\begin{gathered} 2x^2+3(x^2+4x+4)=140 \\ 2x^2+3x^2+12x+12=10 \\ 5x^2+12x+12=140 \\ 5x^2+12x+12-140=0 \\ 5x^2+12x-128=0 \end{gathered}`
`\begin{gathered} (x-4)(5x+32)=0 \\ x-4=0 \\ x=4 \\ OR \\ 5x+32=0 \\ 5x=-32 \\ x=-(32)/(5)=-6.4 \end{gathered}`

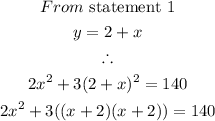
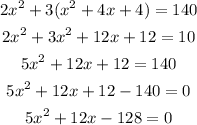
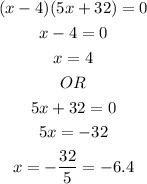
To find y, we would use x =4, because the statement said the numbers are whole numbers

`\begin{gathered} y=2+x \\ where\text{ x=4} \\ y=2+4=6 \\ \end{gathered}`

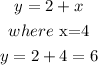
Hence, the numbers are 4 and 6