Question

How many different 4-digit personal identification numbers are possible if no digit can be used twice? a. 1,000 b. 5,040 c. 9,000 d. 10,000

Answer 1

The answer is B. 5,040

Answer 2

5040

Explanation:

To Find : How many different 4-digit personal identification numbers are possible if no digit can be used twice?

Solution :

Since we are given that no digit can be used twice

SO, when one digit is used so next digit will be chosen from the remaining digit an so on .

the digits can be 0,1,2,3,4,5,6,7,8,9

Since these are 10 in number

out of these 10 we need to choose 4 but no digit can be used twice

⇒
`_(10)\textrm{C}_1 *_(9)\textrm{C}_1 *_(8)\textrm{C}_1*_(7)\textrm{C}_1`

Formula :
`_(n)\textrm{C}_r=(n!)/(r!(n-r)!)`

thus using this formula

⇒
`(10!)/(1!(10-1)!) *(9!)/(1!(9-1)!)*(8!)/(1!(8-1)!)*(7!)/(1!(7-1)!)`

⇒
`(10!)/(9!) *(9!)/(8!)*(8!)/(7!)*(7!)/(6!)`

⇒
`10*9*8*7`

⇒
`5040`

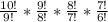
Thus different 5040 number of 4-digit personal identification numbers are possible if no digit can be used twice