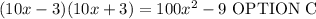Question

the area of the rectangular rug is represented by expression (10x-3)(10x+3) square units. Which expression represents an equivalent area in square units?

Answer 1

- The question would like us to expand the brackets in the expression below:

`(10x-3)(10x+3)`

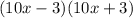
- We can proceed to expand this normally, but instead of going through the tedious process of doing that, we can simply observe that this question is a scenario of a "Difference of two squares". "Difference of two squares" is defined below:

`(A+B)(A-B)=A^2-B^2`

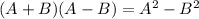
- In this case, we can think of it like this:

`\begin{gathered} A=10x \\ B=3 \end{gathered}`

- Thus, we can easily expand this expression as follows:

`\begin{gathered} (10x-3)(10x+3)=(10x)^2-(3)^2 \\ \\ \therefore(10x-3)(10x+3)=100x^2-9 \end{gathered}`

Final Answer

The answer is

`(10x-3)(10x+3)=100x^2-9\text{ \lparen OPTION C\rparen}`