Question

Solve the compound inequality. 3x+1>2x−3>x−12

Answer 1

We have to solve this inequality.

We divide this inequality at each of the signs and solve for the two boundaries of x.

`\begin{gathered} 3x+1>2x-3 \\ 3x-2x+1>2x-3-2x \\ x+1>-3 \\ x>-3-1 \\ x>-4 \end{gathered}`

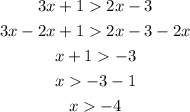
We then use the other inequality and solve for x:

`\begin{gathered} 2x-3>x-12 \\ 2x-x-3>x-x-12 \\ x-3>-12 \\ x>-12+3 \\ x>-9 \end{gathered}`

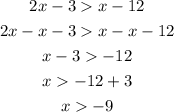
This last result, x > -9, is included in the previous result, as x has to be greater than -4.

We can test the result for x = 0, which should satisfy both equations:

`\begin{gathered} 3\cdot0+1>2\cdot0-3>0-12 \\ 1>-3>-12\longrightarrow\text{True} \end{gathered}`

If x is less or equal than -4, the right side of the inequality won't be satisfied.

If x is less or equal than -9, both sides of the inequality are not satisfied.

Answer:

The interval that is a solution for the compound inequality is x > -4