Question

can you please help me answer part A and B and C of question number 4 please answer clear and in detail it would be appreciated ASAP thank you very much

Answer 1

Given the pyramid with a square base in the image, the following are the solution steps wo find the surface area.

Step 1: Describe the formula of the surface area of the pyramid

The pyramid consists of a square base and 4 triangles, therefore the surface area of the pyramid will be the area of the square base added to the areas of the 4 triangles.

Step 2: To get the surface area of the pyramid, we calculate the area of the square base

`\begin{gathered} \text{For the square base} \\ \text{Area}=s^2,s=25\text{ f}eet \\ \text{Area}=25^2=625feet^2 \end{gathered}`

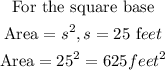
Step 3: We calculate the area of the 4 triangles

`\begin{gathered} \text{Area}_{\text{triangle}}=(1)/(2)bh,\text{ b=25}feet,h=32 \\ \text{Area}_{\text{triangle}}=(1)/(2)*25*32 \\ \text{Area}_{\text{triangle}}=25*16=400feet^2 \\ \text{Area of 4 triangles will be 4}*\text{Area}_{\text{triangle}} \\ \text{Area}_{\text{all traingles}}=4*400 \\ \text{Area}_{\text{all traingles}}=1600feet^2 \end{gathered}`

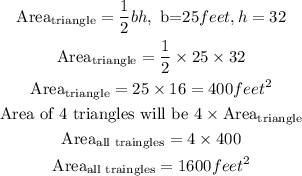
Step 4: Calculate the surface area by following the instruction in Step 1:

`1600+625=2225feet^2`

Hence, the surface area of the pyramid equals 2225 square feet.

Question b: Surface area of the metal soup can

The metal soup can has the shape of a cylinder, therefore the surface area will be:

Hence, the surface area of the metal soup can equals 785.39816 square cm.

Question c: Surface area of the ice cream cone with an open top

It can be seen from the image that the height is missing from the cone.

Surafce area of a cone with an open top means we exclude the area of the circular surface:

Hence, the surface area of the ice cream cone can equals 245.107 square cm.