Question

There are two parts to this question. A) The exponential growth function that models the data is A=

Answer 1

`\begin{gathered} (a)A=5.86e^(0.01t) \\ (b)Year\;2100 \end{gathered}`

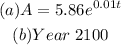
Explanation:

The exponential growth model is given as:

`A=A_oe^(kt)`

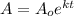
The population in Year 2000 (t=0) = 5.86 million.

`\begin{gathered} 5.86=A_0e^(k(0)) \\ \implies A_0=5.86 \end{gathered}`

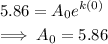
The projected population in Year 2015 (t=15) = 7 million.

`\begin{gathered} A(15)=5.86e^(15k) \\ 7=5.86e^(15k) \end{gathered}`

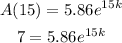
We solve the equation for k.

`\begin{gathered} \text{ Divide both sides by 5.86} \\ (7)/(5.86)=e^(15k) \\ \text{ Take the natural logarithm \lparen ln\rparen of both sides:} \\ ln((7)/(5.86))=ln(e^(15k)) \\ \ln((7)/(5.86))=15k \\ \text{ DIvide both sides by 15} \\ k=(1)/(15)\ln((7)/(5.86)) \\ k\approx0.01 \end{gathered}`

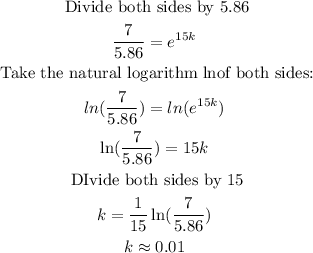
(a)The exponential growth function that models the data is:

`A=5.86e^(0.01t)`

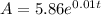
(b)When the population is 16 million, i.e. A=16, we want to find the value of t.

`\begin{gathered} 16=5.86e^(0.01t) \\ \text{ Divide both sides by 5.86} \\ (16)/(5.86)=e^(0.01t) \\ \text{ Take the }\ln\text{ of both sides} \\ \ln((16)/(5.86))=\ln(e^(0.01t)) \\ \ln((16)/(5.86))=0.01t \\ \text{ Divide both sides by 0.01} \\ t=(1)/(0.01)\ln((16)/(5.86)) \\ t\approx100.44 \end{gathered}`

Add 100 to the year 2000:

`2000+100=2100`

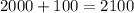
The population will be 16 million in the year 2100.