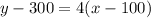Question

After the drama club sold 100 tickets to a show, it had $300 in profit. After the next show, it had sold a total of 200 tickets and had a total of $700 profit. Which equation models the total profit, y, based on the number of tickets sold, x?

a. y – 300 = 4(x – 100)
b. y + 300 = 4(x + 100)
c. y + 300 = 2.5(x + 100)
d.y – 300 = 2.5(x – 100)

HELPPPPPPPPPPP

Answer 1

the answer to this problem is a. y-300=4(x-100)

Answer 2

Option a :
`y-300 = 4(x - 100)`

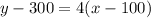
Explanation:

Given :

The drama club sold 100 tickets to a show, it had $300 in profit.

The next show, it had sold a total of 200 tickets and had a total of $700 profit.

To Find : Equation models the total profit, y, based on the number of tickets sold, x

Solution :

For 100 tickets he had $300 in profit .

⇒ (
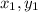`x_(1) ,y_(1)`)=(100,300)

For 200 tickets he had $700 in profit .

⇒ (
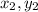`x_(2) ,y_(2)`)=(200,700)

We will use point slope form i.e.

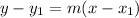`y-y_(1) = m(x - x_(1))` --(A)

Now, to calculate m we will use slope formula :

`m = (y_(2) -y_(1) )/(x_(2)-x_(1)  )`

`m = (700 -300)/(200-100)`

`m =4`

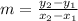
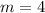
Now, putting values in (A)

`y-300 = 4(x - 100)`

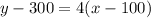
Thus Option a is correct i.e.
`y-300 = 4(x - 100)`