Question

Find the system of inequalities representing the given situation?

Answer 1

The question was written by the student in a comment because the image contains no question at all.

• Kathy sells candles for $3 and flowers for $5. She plans to sell at least 200 items and likes to earn a minimum of $2500.

Answer:

`\left\{\begin{matrix}x + y = 200\\ 3x + 5y = 2500\end{matrix}\right`

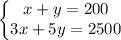
Explanation:

System of equations

Kathy sells candles for $3 and flowers for $5. Let's set the following variables:

x = number of candles Kathy sells

y = number of flowers Kathy sells

She plans to sell 200 items, thus:

x + y = 200

She also likes to earn $2,500. The equation for this condition is:

3x + 5y = 2500

The system of equations is

`\left\{\begin{matrix}x + y = 200\\ 3x + 5y = 2500\end{matrix}\right`