Since the y-intercept of the polynomial must be 3600, then:
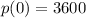
Since the only zeroes of the polynomial must be x=5, -4, -6 and 1, then the factors of the polynomial, are:
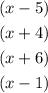
Let the multiplicity of the factor (x-1) be equal to 3 and let the multiplicity of the rest of the factors to be equal to 1. Then:
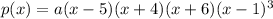
Where a is a constant. Notice that the degree of that polynomial is 6. Evaluate it at x=0 to find the value of a that makes the y-intercept to be equal to 3600:
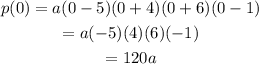
Since p(0)=3600, then:
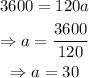
Therefore, the following polynomial is a 6th degree polynomial with zeroes at the values of 5, -4, -6 and -1 with a y-intercept equal to 3600:
