Question

Line m is parallel to line n. Find the value of x and each missing angle measure. Explain.

Answer 1

We want to find the missing angles below

Angle 1 and 86 degree makes a straight-line, so

`\begin{gathered} \text{angle 1 + 86}\degree=180\degree \\ \text{angle 1 = 180 - 86} \\ \text{angle 1 = }94\degree \end{gathered}`

Angle 2 is vertically opposite to 86 degrees,

Hence angle 2 is 86 degrees

Angle 3 is vertically opposite to angle 1, so

Angle 3 is 94 degrees

angle 6 is corresponding to angle 3,

So angle 6 is 94 degrees

Angle 4 is vertically opposite to angle 6

Hence angle 4 is 94 degrees

Angle 5 is corresponding to 86 degrees

Hence angle 5 is 86 degrees

Angle 5 is vertically opposite to

`\begin{gathered} (2x+25)\degree \\ \text{But angle 5 = 86}\degree \\ So \\ (2x+25)\degree=86\degree \\ 2x+25=86 \\ 2x=86-25 \\ 2x=61 \\ x=(61)/(2) \\ x=30.5 \end{gathered}`

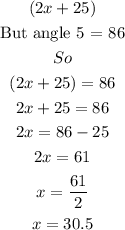
Hence x is 30.5